How to read virtual sundials

How to read the time

Basic principles: Please read the hour scale indicated by the shadow extending from the center of the dial face. In the example in Fig. 1, the time is exactly 8 o'clock.

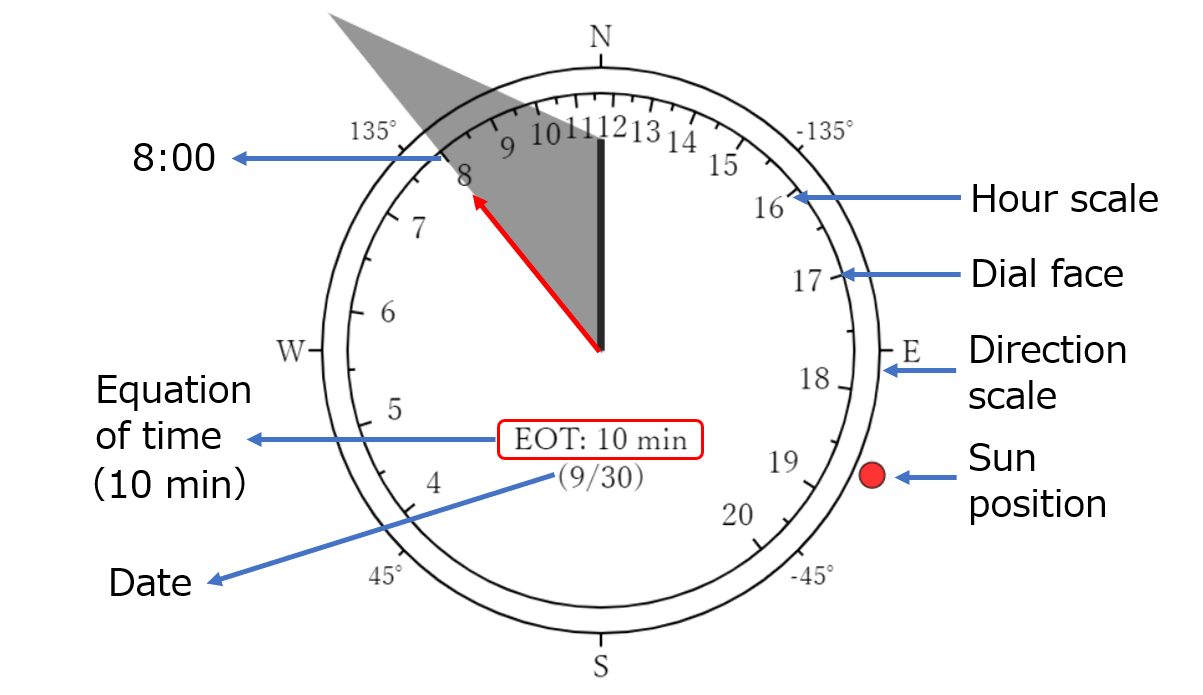
To know more accurate time: Please subtract the equation of time (EOT) from the time indicated by the shadow. The EOT is displayed on the dial face. In the example in Fig. 1, the time indicated by the shadow is 8:00 and the EOT is 10 minutes. Therefore, 8:00 - 10 minutes = 7:50. In the case that the EOT is a negative value (e.g. -10 minutes), the negative value is subtracted. This means that the numerical part is added (e.g. 8:00-(-10 minutes) = 8:10).

The time displayed digitally at the top of the sundial is not the time indicated by the sundial, but the exact time your device has (it is not shown in the figure above). Please try to compare it with the time you read on the sundial.

Equation of time (EOT)

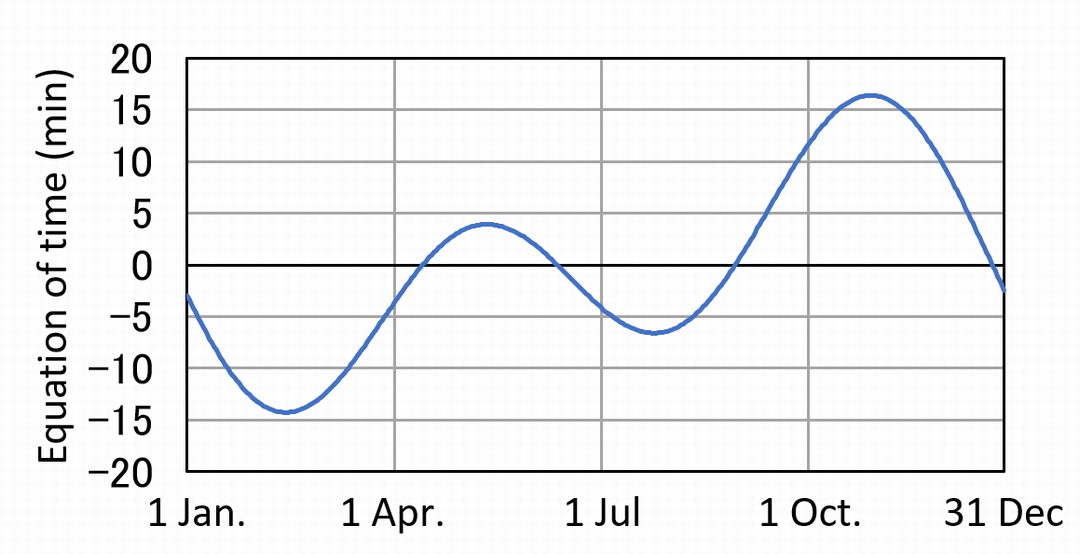
This sundial shows a time scale corrected for the time difference caused by longitude. However, the sundial has seasonal errors that cannot be corrected. This is because the time the sun takes to reach the south is not constant throughout the year. The variation in time is known as the equation of time (EOT). The EOT varies in the range of about -15 to +16 minutes. Fig. 2 shows the variation of the EOT throughout the year. Note that the + and - signs of the EOT can be reversed depending on the material.

The EOT occurs because the Earth's orbit is elliptical and the Earth's axis of rotation is not perpendicular to the plane of revolution. If you want to know more details, please see the Wikipedia.

Display of sun position

This sundial also shows the position (azimuth) of the sun. The small ○ mark on the outer circle of the sundial indicates the position of the sun (Fig. 3). The sun is displayed in red from sunrise to sunset and in grey from sunset to sunrise.

If you place the phone on a horizontal surface, display the virtual sundial in real-time mode, and turn the ○ mark in the direction of the real sun, the shadow of the virtual sundial will match the real sundial. At this point, the sundial can also be used as a compass, as the direction of the dial is the same as the direction at that location.

In addition, the sun's altitude (elevation angle from the horizon to the centre of the sun) and azimuth (south: 0°, west: 90°, east: -90°, north: ±180°) are digitally displayed on the lower right-hand side of the sundial.

Sun altitude and sunrise/sunset

This sundial shows shadows when the sun's altitude is above -0.265°. As shown in Fig. 4, the Sun's diameter is about 0.53°。

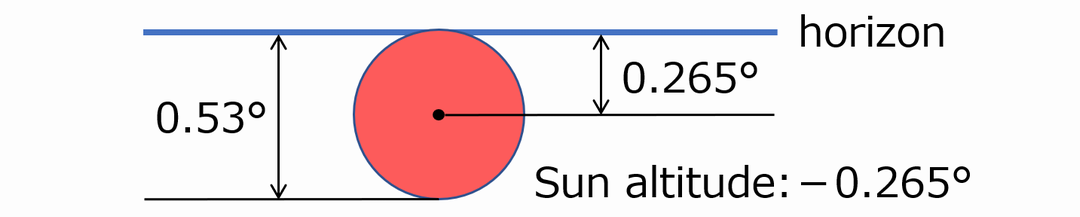
Sunrise/sunset is defined as the time when the upper part of the sun reaches the horizon. As this programme measures altitude from the centre of the sun, it will be judged as sunrise if the altitude of the sun is above -0.265° (and as sunset if it is less than -0.265°). In addition, when the sun is close to the horizon, i.e. the altitude of the sun is low, the sun will appear higher than it actually is due to the influence of the atmosphere. The calculation of this sundial also takes this effect into account.

Sundial design

The sundial shown on this website is a type called a "horizontal sundial", which is installed so that the face is horizontal. If the basic rules are followed, the sundial can be made in various designs. The design used on this website is shown in Fig.5. This sundial uses a circular dial and a right-angled triangular gnomon (a plate that casts a shadow). The origin of the gnomon is set at the centre of the circular dial and the length of the gnomon axis is equal to the radius of the dial.

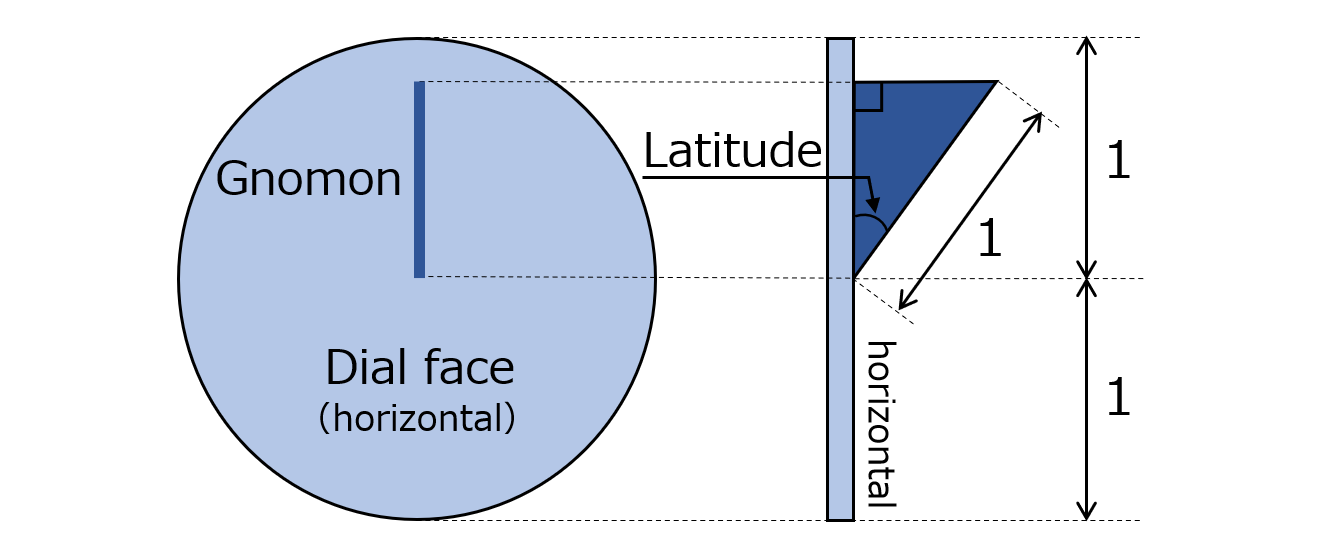
• It is difficult to tell the exact time at low latitudes (near the equator) using this type of sundial, because the intervals between the hour scales are narrow. And this sundial doesn't work at the equator (0 degrees latitude), because the height of the gnomon is zero and no shadow is created.
• Since the azimuth cannot be defined at latitude = ±90°, when ±90° is set, the calculation is made by considering ±90° as ±89.99°.
• Fig. 5 shows the gnomon as if it had a certain thickness, but the thickness is ignored in the calculation. The shadow is shown assuming that the thickness of the gnomon is zero (extremely thin).

Sundial accuracy

Please note that even after correcting the equation of time (EOT), there may still be a time error of up to several minutes. This is because the calculation of the sun's position is not perfect. At the top of the sundial, the exact time obtained from your device is displayed so you can check the accuracy of the time.

How to simulate a sundial

A simple description of how to calculate and display a sundial is as follows.

(1) Determine the shape of the gnomon from the latitude and display the line of the gnomon on the dial. (2) Calculate the angles of the hour scales from the latitude, longitude and time zone, and display the scales on the dial. (3) Calculate the position of the sun (altitude and direction) from the latitude, longitude, date, time and time zone. (4) Calculates the shape of the shadow from the position of the sun and the shape of the gnomon, and display it.

The real-time version recalculates and updates the display every 2 seconds.